Problems solving 1D: Resonance frequency of column (Mono-lithology)
A column of elastic material (single-lithology) resting on a rigid base has natural periods of vibration that depend on the mode of oscillation. The theoretical value for natural frequency of oscillation (f) is given by:
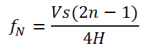
where:
- H = column height of soil (for this verification H=8.0 m)
- Vs = velocity of shear waves (for this verification Vs=100 m/s)
- T = natural period of oscillation (for this verification T=0.32 s --> F=3.125 Hz)
Geometry and Material Properties
The model is a column whit dimension 8.0x1.0 meters and it make of eight (8) regular elements and eighteen (18) nodes.

Material Properties
| Density | Wave Vs | Shear Modulus | Poisson Ratio | Damping |
|---|---|---|---|---|
| 10 N/m2 | 100 m/s | 1.019E4 N/m2 | 0.10 | 1.0 % |
Boudary Conditions
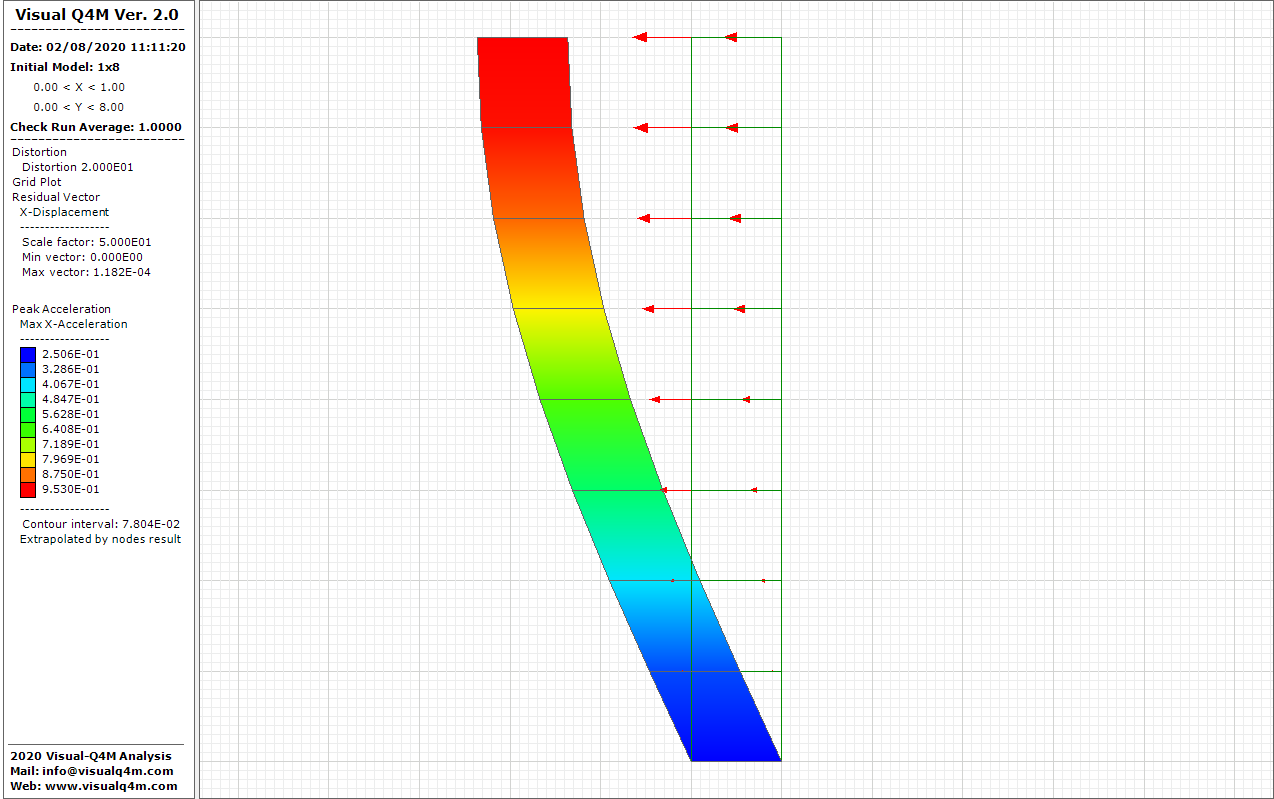
The column have a behavior in shear and the vertical motion (Y) is inhibited to eliminate bending modes. The loading is applied to the base (XY).
You apply the load horizontal component only in the nodes with X-Direction locked:
- Node 1, 1 --> Fix XY-Direction
- Node 2, 1 --> Fix XY-Direction
Input Motion
The horizontal acceleration (max 0.2g) given by:
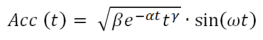
where:
- [α] = 2.500
- [β] = 0.011
- [γ] = 8.000
- [ω] (angular frequency) = 2πf
- f = Variable Frequency (1.000, 2.000, 3.000, 4.000, 5.000, 6.000 Hz)
Results
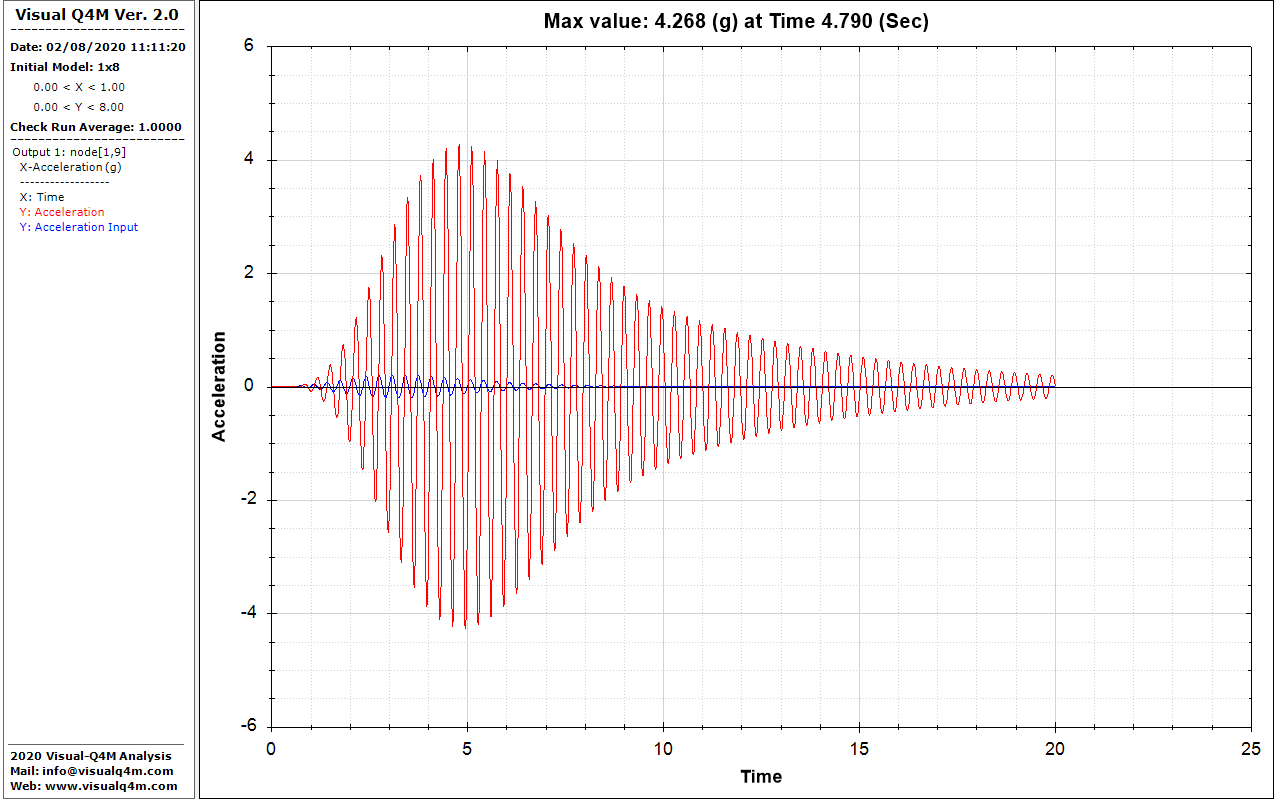
It is shown the maximum amplification obtained when the frequency of the input motion is near to the resonance frequency of the column. The figures show the horizontal acceleration at the top of the column (node 1,9) as a function of time and the acceleration spectrum with a predominant period of 0.325 sec (f = 3.077 Hz).
-

- Input Acceleration Spectrum
-

- Output Acceleration Spectrum
Amplification Factor
| Frequency Input Motions | 1.0 Hz | 2.0 Hz | 3.0 Hz | 4.0 Hz | 5.0 Hz | 6.0 Hz |
|---|---|---|---|---|---|---|
| Output FHv [0.1 - 2.50 Sec] | 1.136 | 1.873 | 20.530 | 2.302 | 1.215 | 1.003 |
| Output FHa [0.1 - 0.50 Sec] | 1.144 | 1.919 | 20.963 | 2.305 | 1.213 | 1.004 |